The greatest shortcoming of the human race is our inability to understand growth

“For what do we need mathematics in real life?” I don’t even dare to ask how often students ask this question in their school career. As adults we do know that to describe concepts like signal transmission via radio waves or three phase electrical currents engineers need complex mathematics (pun intended). But for our daily life we¹ don’t need anything more complex than the rule of three, do we? For the rest we stick to making jokes about our math teachers telling us we would not have calculators in our pockets everywhere we go.

Jokes aside, I think that this common view on math comes from a failure in our school education. We learned math for computers, which isn’t a common occupation anymore. The downside is, that many important mathematical concepts are not well understood in the general public’s awareness. One of those concepts is growth. For example, we have seen how often politicians and press commentators have misunderstood and still misunderstand exponential growth of Covid-19 cases.

But we can find this even in more “mundane” discussions. My motivation for writing this post is a quote from a video by Youtuber AdamSomthing. At the linked part of the video he says (emphasis by me):

That’s because the heavier the vehicle, the more damage it does to the road; exponentially so.

He than proceeds to show a table where the damage done to a road grows quadratically² by the vehicle’s weight. For most people this looks like a minor detail, but—probably because of the pandemic—I am a little bit iffy about this. So if I have not scared you off by now, let’s strap in for an extensive discussion why understanding growth is an important thing.

The Paper Stacking Thought Experiment

Let’s start with a thought experiment and assume we want to stack sheets of paper, each 0.1mm thick, to reach the moon. For simplicity reasons, we assume the moon is exactly 300000km away from earth’s surface and we stand directly below it³. How many sheets of paper do we need to stack?

Easy enough: 300000km / 0.1mm = 3 trillion. We need some unit conversion and the result is what some mathematicians would call a large-ass number. Most people do not grasp the difference between a million and a billion, let alone a trillion. But other than that, we just need simple linear arithmetic. The vast majority of children can do that after finishing elementary school, so linear growth falls into the “up to the rule of three” category mentioned above.

Let’s change the rules of the thought experiment a little. Now it’s a race. A vast number of people compete in paper stacking to the moon, but they are all equally fast. Since this is a rather boring race, some participants try get an advantage. After stacking the first sheet, two people find out, there is nothing in the rule against cooperating. So they merge their stacks and each put another sheet on top. A third person notices this and merges their stack as well and all three of them add another sheet. This continues on, i.e. each time the team members each add another sheet, another person joins them.

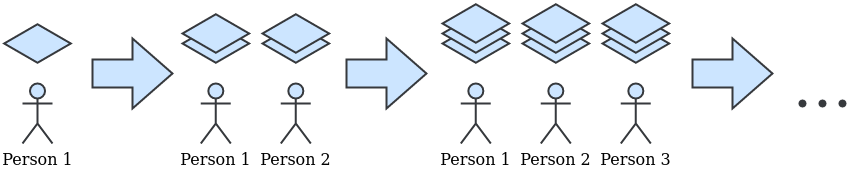
How many people need to join the team to reach the moon? We can see that the number of sheets each person stacks is equal to the number of people, which means our paper stack grows quadratically. To answer the question straight-forward we can setup a quadratic equation:

1. 0.1mm × x² = 300000km | /0.1mm
2. x² = 3 trillion | √
3. x = √(3 trillion) ≈ 1.73 million

Simplifying Mathematics in Everyday Life

The quadratic version of this though experiment is a little bit more tricky than the linear one we saw at the beginning. People experienced with computing in their head might have jumped directly into equation 2, but many people are probably out of their mathematical comfort zone. Quadratic equations are arguably more complicated than the rule of three.

But even though I do believe that many people feel uncomfortable with this math, I do not believe they throw away all their knowledge about it once they finish school. When they need to access this knowledge, they will know at least the rough gist and apply some “common sense” to make a decision.

It is a little bit like the “system 1” and “system 2” thinking described by Daniel Kahneman. This theory has been criticized for lacking scientific rigor, but there are still some very plausible points in it. In short it states that people often don’t make decisions by consciously analyzing the problem and running the numbers (system 2), but by relying on emotion and experience (system 1). And it works, e.g. if I just grab the store brand of a type of cookie I will most certainly save money compared to the name brand, even if I don’t calculate the price per gram.

So why not apply this approach to understanding growth? Many people who can’t solve quadratic equations anymore do still understand that some things grow faster and some things slower than linear. This describes three categories. Let’s call them linear, super-linear and sub-linear. Should be useful enough, shouldn’t it?

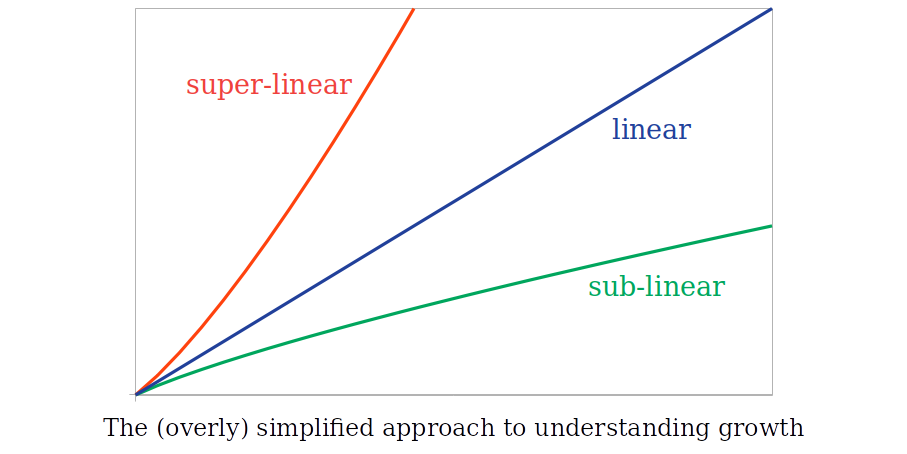
When AdamSomething used the word “exponentially” he probably just wanted to drive home that 4000 pounds of car causes almost 1500 times the amount of road damage 4000 pounds of ridiculously heavy people-bike-combos do. This simplified information is very useful, we can derive a lot from that. We understand why bike infrastructure is so much cheaper than car infrastructure and that replacing two ton SUVs with two ton SUVs with an additional half ton of battery might not be the smartest move, when it comes to infrastructure cost.

Exponential Growth

So if this “short-hand reasoning” is so useful, why am I so hung up on the fact AdamSomething used the word “exponentially”? Yes, technically “exponentially” means something like 2^x and the actual quadratic growth is something with x². But there are exponents in both of those expressions so both of them are somehow “exponential” and both of them grow super-linear. That’s the point, isn’t it?

To answer this question, let’s look at a third version of our paper stacking thought experiment. This time we assume that instead of stacking the paper, we can fold it as often as we like, doubling its thickness⁴ each time. How many times do we have to fold it so it reaches the moon?

The equation we need to solve is: 0.1mm × 2^x = 300000km

To solve it exactly we need to apply those pesky logarithms some people dread to this day. I don’t want to scare away the last people who made it this far into the post and we need a whole number answer anyway to make sense, so I’ll just say it. We need to fold it 42 times⁵. If you don’t believe me, you can easily calculate the left-hand side of the equation for 41 and 42. With 41 we still fall short, reaching about 220000km. With 42 we get to about 440000km, which actually does not only reach the moon, but shoots past it by about 40 times its diameter. So while quadratic growth is considerably faster than linear growth, exponential growth is also considerably faster than quadratic growth.

So when AdamSomething called his quadratic growth “exponentially” he mixed up two completely different things. Not by any ill intent, I believe all he did was to compare two rows of his data table with the rule of three. It was clearly super-linear, but that’s a complicated word I just made up. People have heard “exponential” and after the pandemic it does sound bad, so why not just use it? It’s just a video about traffic infrastructure, after all.

The Downside of Simplification

The problem is, that if some untrue facts are used in a public space—be it a newspaper or a Youtube channel with a million subs—they tend to stick in peoples’ memories and experiences, influencing their “system 1” decision making. Many people say homeopathy is no big deal. What harm could there be in people swallowing tiny orbs of sugar? Nothing at first, but if you teach people to question the soundness of scientific medicine testing, they might start to question things like vaccines as well.

Now when it comes to the harm of misunderstanding growth, we need to look back at the pandemic. How often have you seen people—especially politicians—look at incidence data and state that the situation is under control? Some even said they don’t see exponential growth, yet. Then a few weeks later, when hospitals are at capacity and strict measures have to be put in place, they shrug and say: “Nobody could have predicted this.” All the while the people who actually predicted it, because they know how exponential growth works, collectively smash their heads onto their desks.

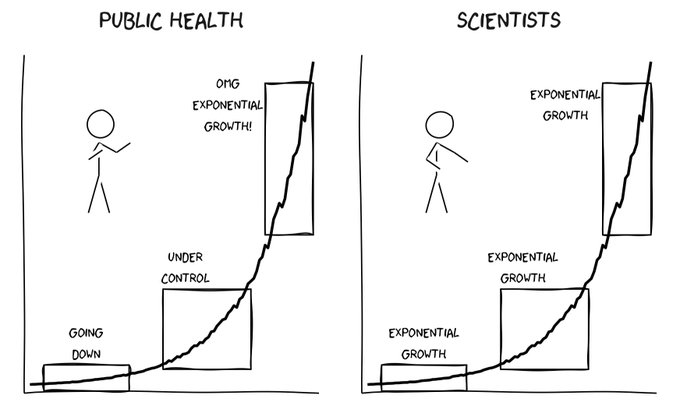
Especially with a lot of noise (“randomness”) it is difficult to tell linear, quadratic and exponential growth apart. But we do know how insufficiently mitigated infections spread and that they do so exponentially⁶. This indicates that even if numbers look manageable now, they will explode in the future.

But politicians don’t decide in a vacuum. During the pandemic everybody and their grandparents had a distinct opinion on how to react properly. Putting reasonable measures in place early when incidence levels were still manageable was highly unpopular. And I dare to wager that a lot of this unpopularity was due to the fact that many people had no idea what was heading our way because they did not understand exponential growth.

Conclusion

Albert A. Bartlett was talking about overpopulation when he said:

The greatest shortcoming of the human race is our inability to understand the exponential function.

As the title of this blog post states, I would argue that it is not just the exponential function, but the mathematical concept of growth as a whole. The often quoted “common sense” is a bad advisor when it comes to these concepts and misunderstanding them can sometimes lead to tangible problems for our society. So if you take just one point home from this long-ass blog post let it be this: It pays to be precise with the wording of your explanations; even in something as mundane as a video about traffic infrastructure.

XKCD style exponential growth picture by Jens von Bergmann